Featuring Professor Volkmar Welker from Philipps-Universität Marburg.
More links & stuff in full description below ↓↓↓
T-Shirt, Mug, Sticker based on this:
Professor Volkmar Welker:
Triangle videos:
Numberphile is supported by the Simons Laufer Mathematical Sciences Institute (formerly MSRI):
We are also supported by Science Sandbox, a Simons Foundation initiative dedicated to engaging everyone with the process of science.
And support from The Akamai Foundation – dedicated to encouraging the next generation of technology innovators and equitable access to STEM education –
NUMBERPHILE
Website:
Numberphile on Facebook:
Numberphile tweets:
Subscribe:
Videos by Brady Haran
Animation by Pete McPartlan
Thanks to the Numberphile Society, especially CJ Smith!
Patreon:
Numberphile T-Shirts and Merch:
Brady’s videos subreddit:
Brady’s latest videos across all channels:
Sign up for (occasional) emails:
✅ Camiseta De Futbol Baratas Player vs Fan | camisetasfutboleses
🛒 Cómprala aquí: https://www.pidecamisetas.com/
📸 Siguenos en Instagram: https://www.instagram.com/msy_es/
📲 WhatsApp: +86 166 5930 6369
👏 ¡Muchas gracias por ver el vídeo! 👏
🔴 LIKE & SUBSCRIBE 🔴
🔴 Keywords:Camisetas UEFA CHAMPIONS
__
🏷️ TAGS:
CAMISETA Real Madrid 22/23
camisetasfutboleses
camisetasfutboleses.com
CAMISETAS FUTBOL SPAIN
CAMISETAS DE FUTBOL
CAMISETAS FUTBOL Baratas
CAMISETAS DE FUTBOL 2020
CAMISETAS DE FUTBOL 2021
CAMISETAS DE FUTBOL 2022
CAMISETAS FUTBOL EQUIPOS
CAMISETAS FUTBOL SELECCIONES
CAMISETAS DE FUTBOL BARATAS
MEJORES CAMISETAS DE FUTBOL
EQUIPACIONES FUTBOL
EQUIPACIONES DE FUTBOL
Otros sitios web de nuestra empresa para que los visite:

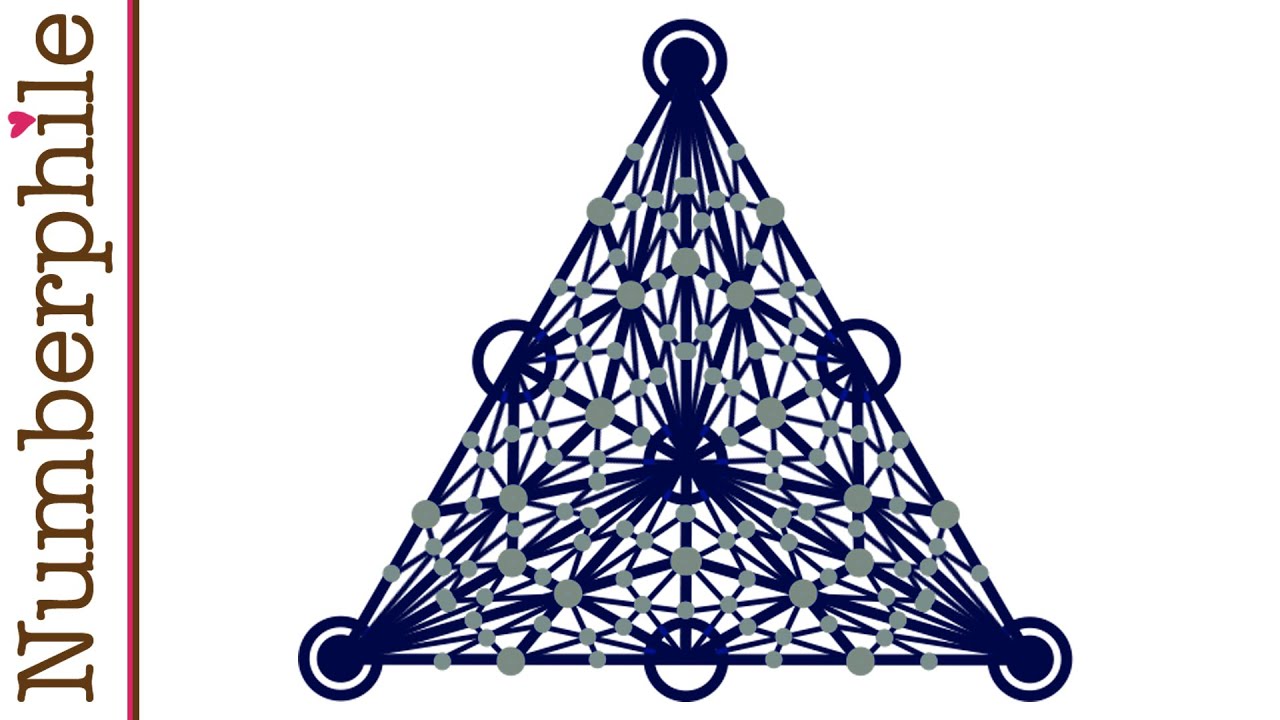
Subdivided Triangle from this week's video looks nice on a tee (or holding tea) – https://numberphile.creator-spring.com/listing/subdivided-tri-numberphile
Truly amazing video Numberphile, fantastic explanation, brilliant work, very informative, very proud of you Numberphile. (:
At a time general constant don't work what is that time?
We can apply it another shapes as well as 3D shapes.
Connect point and lines we get results.
Expand 3D shapes.
Expanding angles rules.
Expanding 3D shapes.
Expanding angles
Expanding triangles rules.
The tetrahedron has several connections that were drawn that don't adhere to the rule of not having a -1 ánd a +1 in the difference between points, so that contradicts what was said.
Also, there are three internal edges that fit the rule of having a difference of 1 (ignoring the above, otherwise there will be none), so how is the single edge chosen? All three are symmetric. If you cut on all of those (you'd have to add a vertex at the intersection) the result is 8 extra pyramids, all nicely symmetric along one of their axes. For subdivision into pyramids this is not necessary, as cutting along a single internal edge as was shown is already enough to result in 4 (albeit asymmetric) pyramids, but it feels like we're missing part of the algorithm here.
EDIT: I was wrong! I forgot to first convert the coordinates to cumulative sums. If you do this, all differences only have -1 or +1 and only one of the internal edges fits the rule, amazing!
What a weird way to say "link every points with distance <2" lol
And then someone's going to try to generalize it. This is very interesting.
What was the point of raising the triangle into the third dimension? It seemed to only complicate things unnecessarily.
I love Numberphile, but this one hurt my brain.
It's Triangles all the way down.
6:00 in and I have no idea wtf the point is in all this
for dimension of 3 also interesting question how to "combine" edges into tetrahedrons.
What is the mortality rate of C-19 ? , is it possible to have all the casualties they said with that rate? Numbers do not match
I would love to hear someone talking on how to subdivide triangles on the surface of a sphere and other noneuclidian planes.
Y’all make a great team. It couldn’t have been achieved with only one of you.
how quad remeshing works?
Tri-force subdivision!
I like the edge-deciding trick for the edgewise subdivision. That'll give me something to think about for a while.
I've got some vague ideas about graph adjacency and Grey codes. I wonder if I can nut it out before I give up and go off reading?
WTF is the point of this video?
100-11-47 How to find
Brady, did you go to Germany to film this? Or was the professor in England (or the US)?
Call me simplistic, but why not just take the midpoints and connect them?
Parker subdivision ends up with squares.
I'm always amazed by the types of questions Brady asks the subject matter expert. It really helps ground the topic, and dig a little deeper than the presenter would have normally delved.
I was left confused about what the point of this was. Could there not have been more context supplied from the beginning about what we were looking at?
1 is such a powerful prime it even chops shapes up.
17,,53
I wish they did a non-equilateral triangle, which he said would work at the beginning, just to get a better visualization of how the congruence builds on the sub-division.
Could you make a video about the practical applications of topology? What have we learned from the study of deeper geometrics, and topology in the past century that we can see in our daily lives?
Very nice work on the animations
I wonder what a Parker subdivision would be. Maybe it would have a double-edge or double-vertex. Maybe it would have a point labeled (-1, 0, 1) or something else not allowed. Maybe it would have a quadrilateral hidden in there. I can't decide.
Better hope the Brady Subdivision doesn't end up being like the Parker Square
I did this type of subdivision. I was trying to recreate Conway's polyhedron operations, but only got to subdividing and expanding.