Learn more complex numbers from Brilliant: 👉 (20% off with this link!)
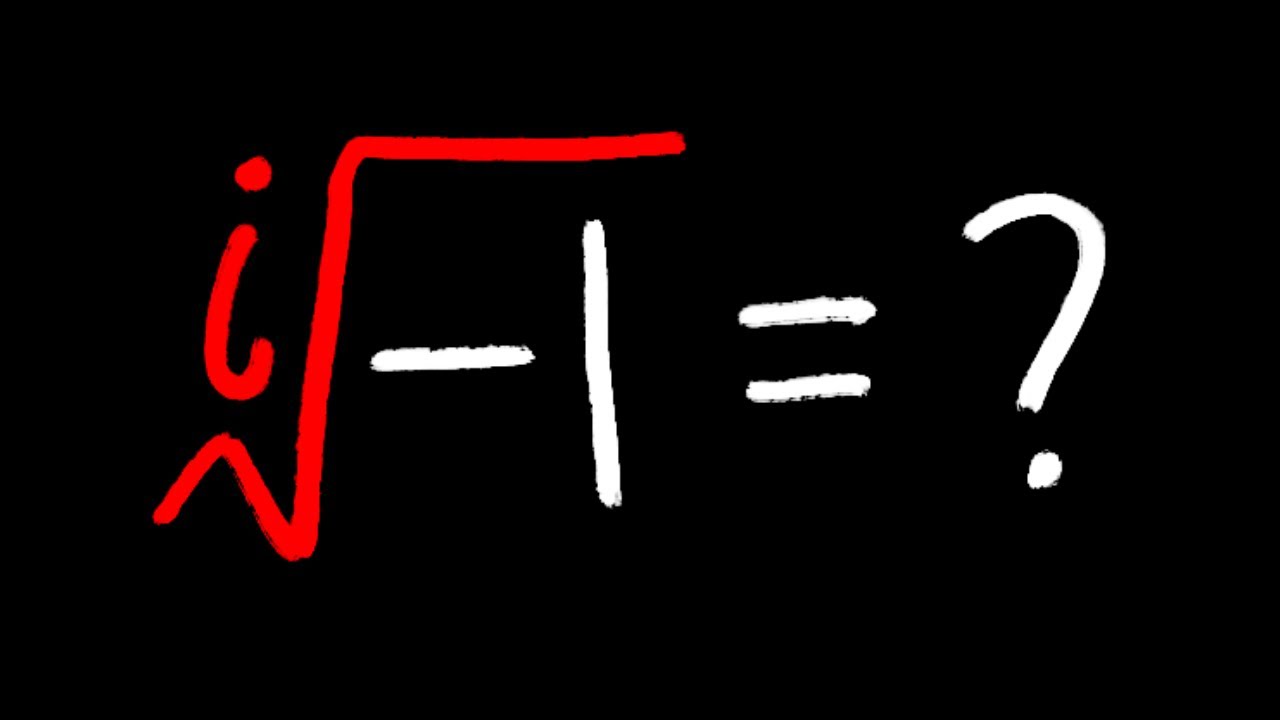
We know the principal square root of -1 is the imaginary unit i but what if we have the i-th root of -1? We will do a quick review on converting a complex number from the standard form a+bi to its polar form re^(i*theta). Then we will see the surprising answer that the i-th root of -1 will actually give us.
Related videos:
the proof of the Euler’s identity (with the Taylor series):
i-th root of i:
🛍 Shop Euler’s Identity t-shirt (as seen in the video):
10% off with the code «WELCOME10»
0:00 what’s the i-th root of -1
5:30 check out Brilliant
6:08 challenging question (-1)^pi
—————————————-
***Thanks to ALL my lovely patrons for supporting my channel and believing in what I do***
AP-IP Ben Delo Marcelo Silva Ehud Ezra 3blue1brown Joseph DeStefano
Mark Mann Philippe Zivan Sussholz AlkanKondo89 Adam Quentin Colley
Gary Tugan Stephen Stofka Alex Dodge Gary Huntress Alison Hansel
Delton Ding Klemens Christopher Ursich buda Vincent Poirier Toma Kolev
Tibees Bob Maxell A.B.C Cristian Navarro Jan Bormans Galios Theorist
Robert Sundling Stuart Wurtman Nick S William O’Corrigan Ron Jensen
Patapom Daniel Kahn Lea Denise James Steven Ridgway Jason Bucata
Mirko Schultz xeioex Jean-Manuel Izaret Jason Clement robert huff
Julian Moik Hiu Fung Lam Ronald Bryant Jan Řehák Robert Toltowicz
Angel Marchev, Jr. Antonio Luiz Brandao SquadriWilliam Laderer Natasha Caron Yevonnael Andrew Angel Marchev Sam Padilla ScienceBro Ryan Bingham
Papa Fassi Hoang Nguyen Arun Iyengar Michael Miller Sandun Panthangi
Skorj Olafsen Riley Faison Rolf Waefler Andrew Jack Ingham P Dwag Jason Kevin Davis Franco Tejero Klasseh Khornate Richard Payne Witek Mozga Brandon Smith Jan Lukas Kiermeyer Ralph Sato Kischel Nair Carsten Milkau Keith Kevelson Christoph Hipp Witness Forest Roberts Abd-alijaleel Laraki Anthony Bruent-Bessette Samuel Gronwold Tyler Bennett christopher careta Troy R Katy Lap C Niltiac, Stealer of Souls Jon Daivd R meh Tom Noa Overloop Jude Khine R3factor. Jasmine Soni L wan na Marcelo Silva Samuel N Anthony Rogers Mark Madsen Robert Da Costa Nathan Kean Timothy Raymond Gregory Henzie Lauren Danielle Nadia Rahman Evangline McDonald
—————————————-
💪 If you would also like to support this channel and have your name in the video description, then you could become my patron here
✅ Camiseta De Futbol Baratas Player vs Fan | camisetasfutboleses
🛒 Cómprala aquí: https://www.pidecamisetas.com/
📸 Siguenos en Instagram: https://www.instagram.com/msy_es/
📲 WhatsApp: +86 166 5930 6369
👏 ¡Muchas gracias por ver el vídeo! 👏
🔴 LIKE & SUBSCRIBE 🔴
🔴 Keywords:Camisetas MUJER
__
🏷️ TAGS:
CAMISETA Real Madrid 22/23
camisetasfutboleses
camisetasfutboleses.com
CAMISETAS FUTBOL SPAIN
CAMISETAS DE FUTBOL
CAMISETAS FUTBOL Baratas
CAMISETAS DE FUTBOL 2020
CAMISETAS DE FUTBOL 2021
CAMISETAS DE FUTBOL 2022
CAMISETAS FUTBOL EQUIPOS
CAMISETAS FUTBOL SELECCIONES
CAMISETAS DE FUTBOL BARATAS
MEJORES CAMISETAS DE FUTBOL
EQUIPACIONES FUTBOL
EQUIPACIONES DE FUTBOL
Otros sitios web de nuestra empresa para que los visite:

Learn more complex numbers from Brilliant: 👉 https://brilliant.org/blackpenredpen/ (20% off with this link!)
x^a = b (a,b,x complex numbers) in general has multiple solutions for x, sometimes infinitely many (e.g. when a=i like in this case). How is "the" answer for b^(1/a) selected?
challenge:i^sqrt(i)^sqrt(2)
红笔黑笔老师居然有英文频道
Why have you stopped making regular videos?
Hello bprp
The answer of minus one to power π
Equal e to power i
But if you do the same with the square root of -1: sqrt(-1) = (exp(i*pi*(1+2n))^0.5 = exp(i*pi*(0.5+n)), and it's either =i or =-i 🤔
Congrats on hitting 1 million subs!!
love your videos 🙂
So technically, I can say that sqrt(-1) is close to positive infinity? Neat. Calculating complex numbers in the real world can give such funny answers
Did this one in my head, lol. Just because of the famous e^(i*pi)=-1, so so the i-root of these gives is e^pi (or e^(pi+2pi*z)
way complex and imaginary problem
AAh!! after thinking about it, this is how you would represent a series or a sequence of real numbers.
Beautiful!! now.. what is the physics related to this number? or what does this mean in real world? thanks. I understand that you are a Math teacher, but as you already know math cannot be separated . that's the reason behind my question.
why r= 1?
bro wtf
Hey bprp, can you solve the integral of (e^x²√(x²-1)+1)/x²-√(x²+1)-1 ?
Hello ! I hope you see my comment
I saw this nice question so that I recommend it
The question is : solve the system of equations
a = exp (a) . cos (b)
b = exp (a) . sin (b)
It can be nicely solved by using Lambert W function after letting z = a + ib
Hope you the best … your loyal fan from Syria
Do i^pie
You lost me at 1:00
Very cool!
Cool!
Hello sir how are you? ,My name is Atiqur Rahman. I am a trader. I want to build up an AI Algorithm trading software system on trading, I have been trying to solve a math calculation on trading for a long time. I request your cooperation in this regard
A haven't seen the video, the answere is 1 right?
Couldn't you also say sqrt(-1) = e^(i(π/2 + 2nπ))
Does anyone know what i-th root of i is?
Isn't there technically only 1 solution to the problem, not infinite? I get that (-1)^(1/i) would have infinite solutions due to the argument being of the form pi + 2n*pi, with n being an integer.
However, since the initial statement used the radical symbol, it means the principal value only, right?
Respect bprp!
I used this method:
(-1)^1/i = -1^-i = -1
can anyone tell me whats wrong?
"we're all adults now, let's use pi". I'm dying xDDD
Thought-road: -1^1/i = i^i = 1
Bro the thing written on your shirt… Prove that
Fun. This time I did it before watching the video 😉
(-1)^π
We know e^(iz)=cos(z) + isin(z)
So
e^(iπ)=-1
Then we elevate it to π
(e^(iπ))^π=(-1)^π
So
(-1)^π=e^(iπ²)
But we know that with complex numbers e^(iz)=e^(i(z+2π))
Then
(-1)^π=e^(i(π²+k2π)), k=0,±1,±2…
In polar form: radius=1 Angle=π²+2kπ rad, k=0,±1,±2…
In binomial form
cos(π²)+isin(π²)
Real answer: i 8 sum π
If a root is a function, then it cannot have infinite results. What you gave is the result to the equation
z^i = – 1
Rather than
z=i th root of – 1
But still, for the rest, interesting!
Don't forget 2i×Peyam.
(-1)^pi = cos(pi^2) + i sin(pi^2) is the principal solution, but I think the general form would be (2n+1)pi^2 inside the cos and sin, n in integers, right?
BlackpenRedPenBluePen . . . YEAH!